To find the area under a function in a given interval you need to find the definite integral of the function in that interval.
For the given function:
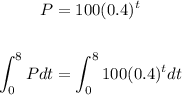
Use the next properties to find the integral:

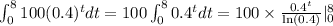
Evaluate the result for the given interval:
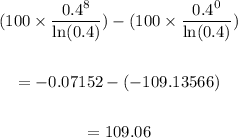
Then, the area under the given function in the interval (0,8) is 109.06