Question

Find the equation line parallel y=(-4/5)x+12 passing through (-6,2)

Answer 1

So we want to find the equation of a line parallel to

`y=-(4)/(5)x+12`

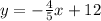
Passing through the point (-6,2).

First, remember that a line is parallel to other if their slopes are the same.

Then, the slope of our parallel line will be also -4/5.

Remember that a line has the following equation:

`y=mx+b`

Where m is the slope and b is the y-intercept.

Now, we know that the parallel line has slope = -4/5 and passes through the point (x,y) = (-6,2), so we could replace in our previous equation as follows:

`\begin{gathered} 2=-(4)/(5)(-6)+b \\ 2=(24)/(5)+b \\ b=2-(24)/(5) \\ b=-(14)/(5) \end{gathered}`

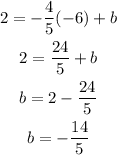
Therefore, the equation of the parallel line to y=(-4/5)x+12 passing through (-6,2) is:

`y=-(4)/(5)x-(14)/(5)`