Here, we want to model an experiment linearlly
From the question, we have it that;
The coordinates are written as;
(number of minutes, mass of gas)
So, what we have to do know is to set up the two given points
These are the points;
(8,302.4) and (17,226.8)
Now, using these two points, we can model the equation
We start by getting the slope of the line that passes through these two points
To do this, we shall use the slope equation
We have this as;
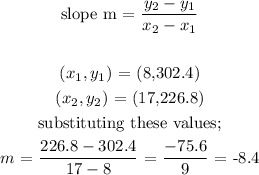
The general equation representing a linear model is ;
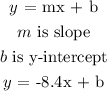
To get the y-intercept so as to write the complete equation, we use any of the two points and substitute its coordinates
Let us substitute the coordinates of the first point
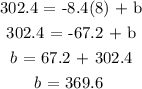
a) Thus, we have the complete linear model as;
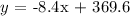
b) To get this, we simply substitute the value of x given into the linear model
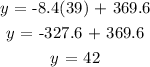
39 minutes after the experiment started, there would be 42 grams
c) If all the gas is gone, then the value of y will br zero at this point
To get the corresponding x-value which is the time, we have it that;
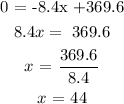
In 44 minutes, all the gas in the container will be gone