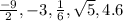Answer:
The number that comes first when the numbers are listed from least to greatest is;
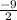
And, arranging the numbers from least to greatest will give;
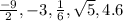
Step-by-step explanation:
We want to arrange the number given below from least to greatest;

From the list of numbers, let us simplify each of them to their approximate decimal.

From the given number, the highest negative number will be the least number.
Because the higher a negative number the lower it becomes.
The highest negative is -4.5 followed by -3.
So, arranging from the least to the greatest we have;
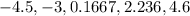
Rewriting it in its initial form we have;
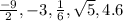
Therefore, The number that comes first when the numbers are listed from least to greatest is;
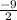
And, arranging the numbers from least to greatest will give;