Question

Enter an equation that passes through the point (12, 7) and forms a system of linear equations with no solution when combined with the equation y=−3/4x+8.

Answer 1

To answer this question, we need to know that two linear equation that does not have solutions must not cross to each other, that is, they do not have a common point. For this case, both lines must be parallel lines. So in the question, we need to find a parallel line to the given line. Two parallel lines have the same slope.

Then, we have that the line must pass through (12, 7), and, because it is parallel to y = -3/4x + 8, and the slope for this line is m = -3/4, then, the line equation is, applying the point-slope form of the line:

`y-y_1=m(x-x_1)`

And

x1 = 12

y1 = 7

m = -3/4

Then

`y-7=-(3)/(4)(x-12)\Rightarrow y-7=-(3)/(4)x+(3)/(4)\cdot12\Rightarrow y-7=-(3)/(4)x+(36)/(4)`
`y-7=-(3)/(4)x+9\Rightarrow y=-(3)/(4)x+9+7\Rightarrow y=-(3)/(4)x+16`

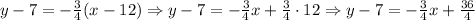
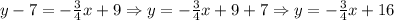
Then, the line equation is y = -3/4 x + 16.

We can check this if we use the elimination method as follows:

This is a FALSE result, and we do not have solutions for this system. Therefore, the line equation is y = -3/4 x + 16.