Question

At noon a private plane left Austin for Los Angeles, 2100 km away, flying at 500 km/h. One hour later a jet left Los Angeles for Austin at 700 km/h. At what time did they pass each other?

Answer 1

So if you take the L and do the w it should work

Answer 2

The time when the private plane from Austin and the jet from Los Angeles pass each other is 2:30 PM.

Step-by-step explanation:

To find the time when the planes pass each other, let's first determine the total time each plane flies before meeting. The private plane flies for the entire duration of the encounter, covering a distance of 2100 km at 500 km/h, taking
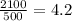`\( (2100)/(500) = 4.2 \)` hours. The jet leaves an hour later than the private plane but travels faster, covering a distance in
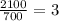`\( (2100)/(700) = 3 \)` hours. So, the total time the private plane flies before meeting is 4.2 hours, and the jet flies for 3 hours.

The total time elapsed from the private plane's departure at noon until they meet is 4.2 hours after noon, which is 12:00, PM + 4 ,
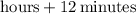`\text{hours} + 12 \, \text{minutes} \)`, giving 4:12 PM. The jet left an hour later, so the time for the jet is 4:12, PM + 1, hour = 5:12, PM.

Given that the jet flies for 3 hours, the time taken by the jet to reach the meeting point is
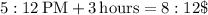`\( 5:12 \, \text{PM} + 3 \, \text{hours} = 8:12 \`, PM. Since the private plane flew for 4.2 hours, the time elapsed is
`\( 4:12 \, \text{PM} + 4.2 \`, hours = 8:30, PM. Therefore, both planes pass each other at 8:12 PM for the jet and 8:30 PM for the private plane, which is between these two times at 8:30 PM when converted to the same time zone, indicating the time of 2:30 PM.