Question

Two friends drive off in different directions from the same place. one heads north at 25 miles per hour, while the other heads east at 40 miles per hour. complete an equation for the distance between the friends after t hours.

Answer 1

Answer:
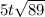`5t√(89)` miles

=======================================================

Step-by-step explanation:

Draw a right triangle. The legs of this triangle represent the directions the two friends travel (one going east, the other going north).

The horizontal leg has side length 40t and the vertical leg has length 25t, where t is the number of hours.

I'm using the idea that distance = rate*time.

We'll use the pythagorean theorem to find the hypotenuse in terms of t.

`a = 40t, \ b = 25t\\\\a^2 + b^2 = c^2\\\\c = √(a^2+b^2)\\\\c = √((40t)^2+(25t)^2)\\\\c = √(1600t^2+625t^2)\\\\c = √(2225t^2)\\\\c = √(25*89t^2)\\\\c = √(25t^2)*√(89)\\\\c = 5t√(89)\\\\`

At time t hours, the distance between the two friends is exactly
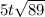`5t√(89)` miles.