Question

Log8 (t) - log8 (5) = 1

Answer 1

t = 40

Explanation:

Given the logarithmic expression:
`log_8 (t) - log_8 5= 1`

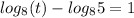
Use the Logarithmic Property (Quotient Rule):

`log_b a - log_b c = log_b ((a)/(c))`

`log_8 (t) - log_8 5 = log_8 ((t)/(5)) = 1`

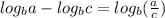
Next, using the Logarithmic Property:
`log_b b= 1`

We must determine the possible value of t that can be divided by 5 to produce a quotient of 8 that will make the logarithmic property,
`log_b b= 1`, true. In that case, t = 40 divided by 5 results in a quotient of 8.

`log_8 (t) - log_8 5 = log_8 ((t)/(5)) = log_8 ((40)/(5)) = log_8 8 = 1`

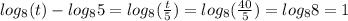
Therefore, the value of t = 40.