Question

a rectangle has a lenght of 33 yard less than 6 times it's width. if the area of the rectangle is 6195 square yards, find the length of the rectangle

Answer 1

The length of the Rectangle is 177 yards

SOLUTION

Problem Statement

The question tells us that the rectangle has a length of 33 yards less than 6 times its width. We are asked to find the length of the rectangle given that the area of the rectangle is 6195 square yards.

Solution

To solve the question, we simply need to interpret each sentence of the question

Let us go through each portion and come up with equations.

`\begin{gathered} Let\text{ the length of the rectangle be }l \\ \text{Let the width of the rectangle be }w \\ \\ \text{ We are told the length is 33 yards times less than 6 times its width: } \\ l=6w-33\text{ (Equation 1)} \\ \\ \text{ We are told that the Area of the rectangle is 6196} \\ \therefore l* w=6195\text{ (Equation 2)} \end{gathered}`

Now that we have the equations, we can solve them simultaneously. We shall use the substitution method.

`\begin{gathered} l=6w-33\text{ (Equation 1)} \\ lw=6195\text{ (Equation 2)} \\ \text{From Equation 2, we have that:} \\ w=(6195)/(l) \\ \text{ Substituting the expression for w into Equation 1.} \\ \\ l=6w-33\text{ becomes,} \\ l=6((6195)/(l))-33 \\ \text{ Multiply both sides by l} \\ l* l=l((6\mleft(6195\mright))/(l)-33) \\ \\ l^2=37,170-33l \\ \text{ rewrite the equation, we have:} \\ l^2+33l-37170=0 \end{gathered}`

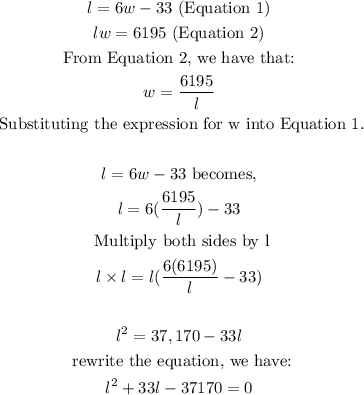
We have obtained a quadratic equation in terms of the length of the rectangle. After solving the equation, we can find the length of the rectangle.

To solve, we shall apply the Quadratic Formula. The Quadratic Formula is given by:

`\begin{gathered} x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ \text{Given the Quadratic equation,} \\ ax^2+bx+c=0 \\ (In\text{ our case, x is }l) \end{gathered}`

Let us apply the formula to our equation as follows:

`\begin{gathered} Given\text{ the equation: } \\ l^2+33l-37170=0 \\ a=1,b=33,c=-37170 \\ \\ \therefore l=\frac{-33\pm\sqrt[]{33^2-4(-37170)(1)}}{2(1)} \\ \\ l=\frac{-33\pm\sqrt[]{1089+148,680}}{2} \\ \\ l=\frac{-33\pm\sqrt[]{149,769}}{2} \\ \\ l=(-33\pm387)/(2) \\ \\ l=177\text{ or -210} \\ \\ \text{ Since we are dealing with lengths and lengths cannot be negative, } \\ \text{Length of the Rectangle is 177 yards} \end{gathered}`

Final Answer

The length of the Rectangle is 177 yards