The solution to the inequality
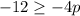 can be represented textually as:
can be represented textually as:
![\[ p \geq 3 \]](https://img.qammunity.org/qa-images/2023/formulas/mathematics/high-school/29yo4w46uijx0x5i9c0n.png)
This means that the set of values for p satisfying the inequality includes all real numbers greater than or equal to 3.
To graph the solution to the inequality
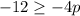 , we can start by isolating p.
, we can start by isolating p.
Divide both sides of the inequality by -4, remembering to reverse the inequality sign when dividing by a negative number:
![\[ -12 \geq -4p \]\[ (-12)/(-4) \leq p \]\[ 3 \leq p \]](https://img.qammunity.org/qa-images/2023/formulas/mathematics/high-school/dp0e7w6s42tfbsaboaxj.png)
Now, we represent this on a number line:
1. Mark a point at 3 on the number line.
2. Draw a solid dot at 3 to include 3 in the solution set because of the inequality sign
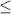 .
.
3. Extend the line to the right, indicating that p can be any value greater than or equal to 3.
The graph on the number line shows that the solution set for p includes all real numbers greater than or equal to 3.