−3x² + 5 = 2x −1
−3x² + 5−(2x −1) = 2x −1 −(2x −1) (Subtract 2x-1 from both sides)
−3x²−2x + 6 = 0
For this equation: a=-3, b=-2, c=6
−3x²+−2x+6=0
===> (Use quadratic formula with a=-3, b=-2, c=6)
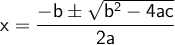
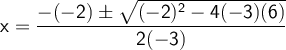
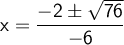

Check intervals in between critical points. (Test values in the intervals to see if they work.)

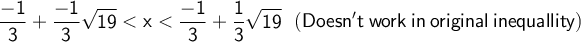


Michael Spymore