Answer:
![\textsf{(a)} \quad \textsf{Interval}=[-10,0].\\\textsf{1.} \quad \textsf{Absolute maximum}=\boxed{497}\\\textsf{2.} \quad \textsf{Absolute maximum}=\boxed{11}](https://img.qammunity.org/2023/formulas/mathematics/college/7wjx06xht2rhrdb9aj0zkqdqu9kbrjvu62.png)
![\textsf{(b)} \quad \textsf{Interval}= [-7, 2].\\\textsf{1.} \quad \textsf{Absolute maximum}=\boxed{445}\\\textsf{2.} \quad \textsf{Absolute maximum}=\boxed{-3}](https://img.qammunity.org/2023/formulas/mathematics/college/3xiebibxfi0v8xqu2jlapkjob83gbn8crr.png)
![\textsf{(c)} \quad \textsf{Interval}=[-10, 2].\\\textsf{1.} \quad \textsf{Absolute maximum}=\boxed{497}\\\textsf{2.} \quad \textsf{Absolute maximum}=\boxed{-3}](https://img.qammunity.org/2023/formulas/mathematics/college/nfe7lxpvcmei4s7z4iwmix8pywmqj80jyv.png)
Explanation:
Given function:
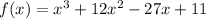
Differentiate the function:
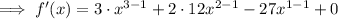

Find the critical points of the function by setting the derivative to zero and solving for x:
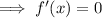
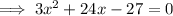
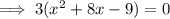
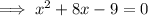
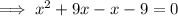
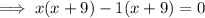
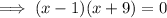
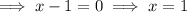
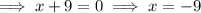
Therefore, the critical points are x = 1 and x = -9.
Evaluate the function at the endpoints of each interval as well as the critical points if the critical points fall within the given interval. The largest and smallest values of the function are the absolute extrema.
Part (a)
Given interval = [-10, 0].
One of the critical points is included in the interval.
Evaluate the function at the endpoints and critical point:
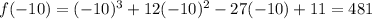
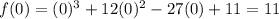
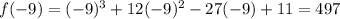
Therefore:
- The absolute maximum of f(x) is 497 which occurs at x = -9.
- The absolute minimum of f(x) is 11 which occurs at x = 0.
Part (b)
Given interval = [-7, 2].
One of the critical points is included in the interval.
Evaluate the function at the endpoints and critical point:

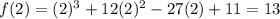

Therefore:
- The absolute maximum of f(x) is 445 which occurs at x = -7.
- The absolute minimum of f(x) is -3 which occurs at x = 1.
Part (c)
Given interval = [-10, 2].
Both of the critical points are included in the interval.
Evaluate the function at the endpoints and critical points:
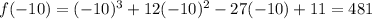
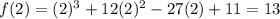
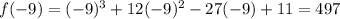

Therefore:
- The absolute maximum of f(x) is 497 which occurs at x = -9.
- The absolute minimum of f(x) is -3 which occurs at x = 1.