Answer:
Choice b: 2 real roots
Explanation:
The original equation is:
x² + 6x = -34
Adding 34 to both sides
x² + 6x + 34 = 0
This is a quadratic equation which can be solved by using the quadratic formula
The roots,
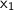 and
and
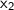 , of a quadratic equation of the form ax² + bx + c = 0 are given by:
, of a quadratic equation of the form ax² + bx + c = 0 are given by:

In the equation given, a = 1, b = 6 and c = 34
So
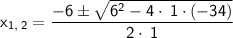
Let's first determine the square root term:
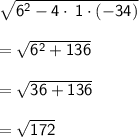
Therefore the two roots are

Both these roots are real and different from each other. There is no need to compute.
Imaginary roots happen only when the term under the square root is negative
So answer is b: 2 real roots