Question

Please solve fast please​

Answer 1

see explanation

Explanation:

Using identities

sec²x = tan²x + 1

cosec²x = cot²x + 1

cos²x =
`(1)/(cos^2x)`

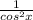
(1)

sin²θ +
`(1)/(sec^20)`

= sin²θ + cos²θ = 1

(2)

9sec²θ - 9tan²θ

= 9sec²θ - 9(sec²θ - 1)

= 9sec²θ - 9sec²θ + 9

= 9

(3)

- 8cot²A + 8cosec²A

= - 8cot²A + 8(cot²A + 1)

= - 8cot²A + 8cot²A + 8

= 8

(4)

- 10cosec²β + 10cot²β

= - 10(cot²β + 1) + 10cot²β

= - 10cot²β - 10 + 10cot²β

= - 10