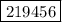Answer:
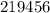
Explanation:
It must end in
 (divisible by 2 rule) and the digits must sum to a multiple of 9(divisible by 9 rule).
(divisible by 2 rule) and the digits must sum to a multiple of 9(divisible by 9 rule).
The digit sum is
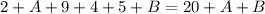 .
.
We want to make
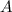 as low as possible because it's a higher digit than
as low as possible because it's a higher digit than
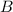 .
.
If
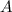 is as low as possible, we have that the sum is
is as low as possible, we have that the sum is
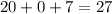 . Uh-oh.
. Uh-oh.
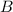 has to be even! So
has to be even! So
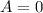 doesn't work.
doesn't work.
If
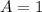 , we have that the sum is
, we have that the sum is
 . Yay!
. Yay!
So, the answer is