Question

Find the length of m

Anybody can help please?

Answer 1

m = 10

Explanation:

We are going to use sine ratio as sine ratio is opposite to hypotenuse.

We know the value of opposite which is 5√3

The value of hypotenuse is m which is unknown.

Therefore:-

`\displaystyle \large{ \sin(60 \degree) = (5 √(3) )/(m) }`

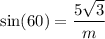
We know that sin60° is √3/2

`\displaystyle \large{ ( √(3) )/(2) = (5 √(3) )/(m) }`

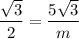
Multiply both sides by LCM which is 2m.

`\displaystyle \large{ ( √(3) )/(2) (2m)= (5 √(3) )/(m) (2m)} \\ \displaystyle \large{ √(3) m=10 √(3) }`

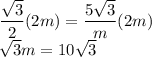
Divid both sides by √3 to isolate m.

`\displaystyle \large{ ( √(3)m )/( √(3) ) = (10 √(3) )/( √(3) ) } \\ \displaystyle \large{ m = 10}`

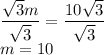
And we're done! The value of m is 10.

Alternative Solutions

If we do not want to use sin60°, we can use cos30°.

Focus the 30°, since for 30°, 5√3 is adjacent and m is hypotenuse.

cosine ratio is adjacent to hypotenuse.

Therefore:-

`\displaystyle \large{ \cos(30 \degree) = (5 √(3) )/(m) }`

We know that cos30° is √3/2

`\displaystyle \large{ ( √(3) )/(2) = (5 √(3) )/(m) }`

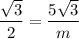
Notice something? Both equations when we use sin60° and cos30° are same. This is called a co-function.

Since sin60° = cos30°, both methods work.

If we do not want to use sin60°, you can use cos30°.