Question

Solve |5x+1|<-2 and write the solution in interval notation.

Answer 1

If you meant to say
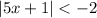`|5x+1| < -2`, then there are no solutions.

This is because the |5x+1| is never negative. This applies to the result of any absolute value function. Absolute value represents distance on a number line. Negative distance is not possible.

---------------------------------------------------------------

If you meant to say
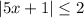`|5x+1| \le 2`, then the steps are shown below

`|5x+1| \le 2\\\\-2 \le 5x+1 \le 2\\\\-2-1 \le 5x+1-1 \le 2-1\\\\-3 \le 5x \le 1\\\\-3/5 \le 5x/5 \le 1/5\\\\-3/5 \le x \le 1/5\\\\-0.6 \le x \le 0.2\\\\`

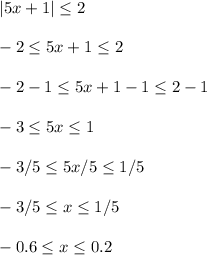
The interval notation would be [-3/5, 1/5] in fraction form

That is equivalent to [-0.6, 0.2] in decimal form.

Use square brackets to include each endpoint.