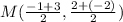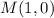Answer: The length of segment
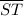 is
is
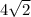 in radical form. I'm unable to see how far to round to, so the decimal form is
in radical form. I'm unable to see how far to round to, so the decimal form is
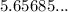 . You will have to round to what the problem asks. The midpoint of the segment is
. You will have to round to what the problem asks. The midpoint of the segment is
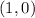 .
.
Explanation:
To find the length of a line segment, use the distance formula
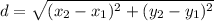 and solve.
and solve.


To find the midpoint of a line segment, use the formula
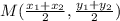 and solve.
and solve.