Question

A cyclist travels 4km in 15 min. What is her speed in m/s?

Answer 1

4.44 m/s

Step-by-step explanation:

Since we want the unit to be in m/s (meter per second), we will be converting from 4 kilometers to meter unit and 15 minutes to second unit.

We know that a kilometer equals to 1000 meters. Multiply both sides by 4:

`\displaystyle{4 \cdot 1 \ \, \sf{km} = 1000 \ \, \sf {m} \cdot 4 }\\\\\displaystyle{4 \ \, \sf{km} = 4000 \ \, \sf {m}}`

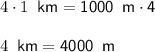
Therefore, 4 kilometers will equal to 4000 meters.

Next, we also know that a minute equals to 60 seconds. Multiply both sides by 15:

`\displaystyle{15 \cdot 1 \ \, \sf{minute} = 60 \ \, \sf {seconds} \cdot 15 }\\\\\displaystyle{15 \ \, \sf{minute} = 900 \ \, \sf {seconds} }`

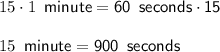
Therefore, 15 minutes will equal to 900 seconds.

Now we have the units in meter and second. We will use the formula of speed to find the rate of change in meter/second. The formula of speed is:

`\displaystyle{v = (s)/(t)}`

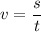
where v is speed, s is distance and t is time. We know that distance is 4000 meters and time is 900 seconds. Substitute in the formula:

`\displaystyle{v = \frac{4000 \ \, \sf{meters}}{900 \ \, \sf{seconds}}}\\\\\displaystyle{v = 4.44 \ \, \sf{m/s}}`

Therefore, her speed is 4.44 m/s