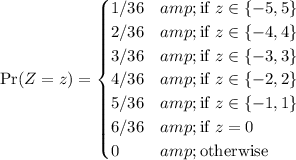and
and
 are independent and identically distributed with PMF
are independent and identically distributed with PMF
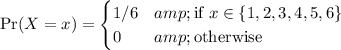
If
 , then
, then
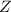 has range/support
has range/support
{-5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5}
where we can get
- -5 = 1 - 6 in 1 way, hence with probability 1/36
- -4 = 1 - 5 = 2 - 6 in 2 ways, with probability 2/36 = 1/18
- -3 = 1 - 4 = 2 - 5 = 3 - 6 in 3 ways, with probability 3/36 = 1/12
and so on, so that the PMF of
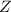 is
is