Since
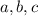 are in geometric progression, if
are in geometric progression, if
 is the common ratio between consecutive terms, then
is the common ratio between consecutive terms, then

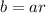

Since
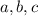 are also in arithmetic progression, if
are also in arithmetic progression, if
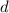 is the common difference between consecutive terms, then
is the common difference between consecutive terms, then


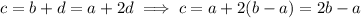
Given that
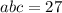 , we have
, we have
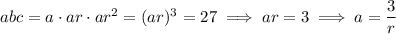
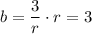
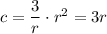
It follows that

Solve for
 .
.
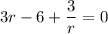
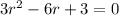
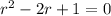
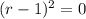

so the only possible sequence is {3, 3, 3, …}.