Answer:
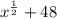
Explanation:
This is hard to explain, but basically you use exponent rules to switch it to an exponent
![(\sqrt[4]{x^(2) +4} )^(3)](https://img.qammunity.org/2023/formulas/mathematics/college/vbstigf8n9ryqjznjgbbczqgr8nytlhkwm.png)
It's easiest if you calculate
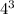 first
first
4³ = 4 x 4 x 4 = 48
Then convert the root to a fraction exponent
![\sqrt[4]{x^(2)} = x^{(2)/(4)} = x^{(1)/(2) }](https://img.qammunity.org/2023/formulas/mathematics/college/qjuj1bqggynyx376kr97hzheseshvqgrpb.png)
Combine them using addition, since addition was in the problem
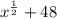
I hope this helps!