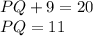Answer: 11 units
Explanation:
We can say that
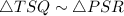 by AA Similarity Postulate. This is since
by AA Similarity Postulate. This is since
 and both ∠TSQ and ∠RSP are right angles, making them congruent.
and both ∠TSQ and ∠RSP are right angles, making them congruent.
Similar triangles have a property that corresponding sides are proportional. Hence, we can say that

We also know that PS is the combined length of PQ and QS. Since we know that QS is 9, let's substitute PQ + 9 in and solve.