Hey there! :)
To find if the solution is a part of the Inequality, we can do by using two methods.
- Solve The Inequality — ( 1 )
- Substitution and Compare — ( 2 )
Solve The Inequality
We are given the Inequality:
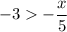
Our goal is to isolate x-term. Since there's 5 as a denominator, what we have to do is to get rid of it by multiplying whole Inequality by 5.
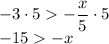
Since -x is equivalent to -1x.
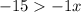
Divide both sides by -1. Do not forget to swap the sign from > to < if we are dividing both sides by negative numbers.
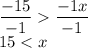
Switch 15 < x to x > 15.

Since x > 15, that means if we substitute x = 10, we'd get 10 > 15 which is false.
Hence, x = 10 is not a part of the Inequality.
Substitution and Compare
From the Inequality, substitute x = 10 in.
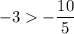
Simplify:
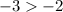
As we know that -3 is less than -2 and not greater. The Inequality is false.
Therefore, x = 10 is not a part of the solution.
Answer
- x = 10 is not the solution to this Inequaliy.