Answer:
115.6° (1 d.p.)
Explanation:
To find the angle between two vectors:
- Create a triangle with the vectors as two sides and the included angle θ between them.
- Find the magnitude of each vector (the length of each side of the triangle).
- Use the cosine rule to find the angle θ.
**Please see attached for the triangle diagram**
Given vectors:
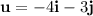
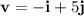
Use Pythagoras Theorem to find the magnitude of each vector:
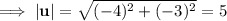
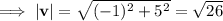
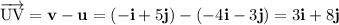

Cosine Rule (for finding angles)
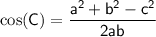
where:
- C = angle
- a and b = sides adjacent the angle
- c = side opposite the angle
Find angle θ using the cosine rule:
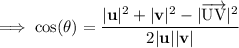

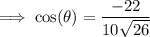


Therefore, the angle between the vectors is 115.6° (1 d.p.).