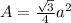Answer:
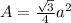
Explanation:
Since an equilateral has all of the sides equal, we can find the height of triangle using:
 . I attached a diagram which should explain how I got the dimensions of the three sides. Using the information from the diagram we get the equation:
. I attached a diagram which should explain how I got the dimensions of the three sides. Using the information from the diagram we get the equation:
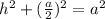
Subtract a^2 from both sides
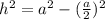
Take the square root of both sides
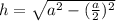
If you know the area of a triangle, it's:
 . In this case the base=a, and the height is what we defined above. Using this we get:
. In this case the base=a, and the height is what we defined above. Using this we get:

We can distribute the exponent over the division to get:
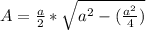
Now we can rewrite a^2 as 4a^2/4
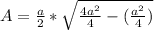
Now add the two fractions:

We can distribute the square root the division just like how we distributed the exponent 2, since the square root can be expressed as an exponent (1/2)
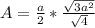
There's a radical identity that states:
![\sqrt[n]{a} * \sqrt[n]{b} = \sqrt[n]{a*b}](https://img.qammunity.org/2023/formulas/mathematics/college/7aol0bbzowc3e009acs2u7qyga9hqpxsk8.png) . We can use this to rewrite one radical as multiple radicals to simplify it:
. We can use this to rewrite one radical as multiple radicals to simplify it:
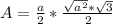
Simplify:
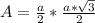
Now multiply the two fractions
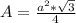
This is the formula for the area of an equilateral triangle, but it is also often written as: