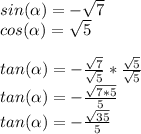Answer:
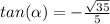
Explanation:
Tan can be defined as:
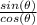 as it simplifies to opposite/adjacent. If you know a bit about the unit circle, you'll know that the x-coordinate is going to be cos(theta) and the y-coordinate is going to be sin(theta). Since the sin(theta) is defined as opposite/hypotenuse, and the hypotenuse = 1, so sin(theta) is defined as the opposite side, which is the y-axis. Same thing goes for cos(theta), except the adjacent side is the x-axis.
as it simplifies to opposite/adjacent. If you know a bit about the unit circle, you'll know that the x-coordinate is going to be cos(theta) and the y-coordinate is going to be sin(theta). Since the sin(theta) is defined as opposite/hypotenuse, and the hypotenuse = 1, so sin(theta) is defined as the opposite side, which is the y-axis. Same thing goes for cos(theta), except the adjacent side is the x-axis.
Using this we can define tan