Question

Assume that the car lot contains 20 percent Lincolns, 45 percent Porches, and 35 percent BMWs. Of the Lincol?

I still can't solve the problem. I did it step by step thoroughly but still get the wrong answer. Can someone help? thanks!

Assume that the car lot contains 20 percent Lincolns,

45 percent Porches, and 35 percent BMWs. Of the Lincolns,

70 percent have two airbags, 60 percent of the Porches have

two airbags, and 90 percent of the BMWs have two airbags. Furthermore,

70 percent of the Lincolns, 40 percent of the Porches, and

30 percent of the BMWs are white. The property of being white is

independent from having two airbags. You are assigned a car at random.

If the car has two airbags and is white, what is the probability that it is a Lincoln?

Answer 1

0.3261 = 32.61% probability that it is a Lincoln

Explanation:

Conditional Probability

We use the conditional probability formula to solve this question. It is

`P(B|A) = (P(A \cap B))/(P(A))`

In which

P(B|A) is the probability of event B happening, given that A happened.

`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

In this question:

Event A: Has two airbags and is white.

Event B: It is a Lincoln.

Probability of a car having two airbags and being white.

This is:

70%*70% of 20%(Lincolns).

60%*40% of 45%(Porches).

90%*30% of 35%(BMWs). So

`P(A) = 0.7*0.7*0.2 + 0.6*0.4*0.45 + 0.9*0.3*0.35 = 0.3005`

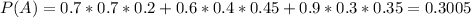
Probability of a car having two airbags and being white, and being a Lincoln:

70%*70% of 20%(Lincolns).

So

`P(A \cap B) = 0.7*0.7*0.2 = 0.098`

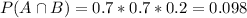
If the car has two airbags and is white, what is the probability that it is a Lincoln?

`P(B|A) = (P(A \cap B))/(P(A)) = (0.098)/(0.3005) = 0.3261`

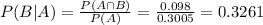
0.3261 = 32.61% probability that it is a Lincoln