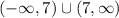Question

When is the equation
`(x-7)/(x^2-4x-21) =(1)/(x+3)` true?

Answer 1

Notice that

`x^2 - 4x - 21 = (x - 7) (x + 3)`

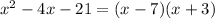
so on the left side, a factor of x - 7 in the numerator and denominator can cancel

`(x-7)/(x^2-4x-21) = (x-7)/((x-7)(x+3)) = \frac1{x+3}`

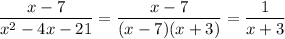
But we can only cancel them out as long as x ≠ 7 (because otherwise we'd have the indeterminate form 0/0 on the left side).

So, the equation is true for all x except x = 7. In set notation,

`\{x \in \mathbb R \mid x \\eq 7\}`

In interval notation,

`(-\infty,7)\cup(7,\infty)`