Question

-3x + 6y = 18

-3x = 6y+14

a) What is the slope of the 1st equation?

b) What is the slope of the 2nd equation?

c) Are they parallel, perpendicular, or neither?

Answer 1

Explanation:

-3x + 6y = 18

-3x = 6y+14

a) the slope of the 1st equation = -(-3)/6 = ½

b) the slope of the 1st equation = -3/6 = -½

c) they are niether parallel nor perpendicular

Answer 2

a) ¹/₂

b) -¹/₂

c) neither

Explanation:

Slope-intercept form of a linear equation:

`y=mx+b`

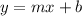
where:

• m is the slope
• b is the y-intercept

Given equations:

`\begin{cases}-3x+6y=18\\-3x=6y+14 \end{cases}`

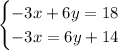
To find the slopes of the given equations, rearrange them to make y the subject then compare with the slope-intercept formula:

Equation 1

`\begin{aligned}-3x+6y & = 18\\6y & = 3x + 18\\y & = (3x+18)/(6)\\\implies y & = (1)/(2)x+3\end{aligned}`

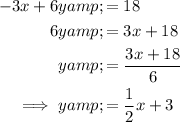
Therefore, the slope of Equation 1 is ¹/₂.

Equation 2

`\begin{aligned}-3x & = 6y+14\\6y & = -3x-14\\y & = (-3x-14)/(6)\\\implies y& = -(1)/(2)x-(14)/(6)\end{aligned}`

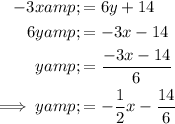
Therefore, the slope of Equation 2 is -¹/₂.

Parallel slopes have the same slope.

Perpendicular slopes are at right angles to each other and therefore the product of their slopes is -1 (negative reciprocals of each other).

Therefore, the slopes of Equation 1 and Equation 2 are neither parallel or perpendicular.