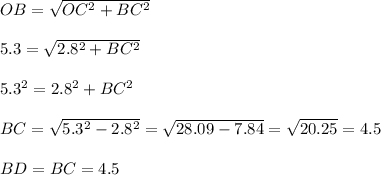Answer:
4.5
Explanation:
First things first - the triangle OCB is similar to ODB - because they are symmetrically reflected over OB. They are also both right triangles, because the tangent is perpendicular to the corresponding radius.
BD = BC
We also know that (because it's a right triangle):