Answer:
The remainder is 11.
Explanation:
We are given the polynomial:
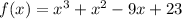
And we want to find its remainder when the polynomial is divided by:
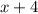
We can use the Polynomial Remainder Theorem. According to the PRT, if we divide a polynomial P(x) by a binomial in the form (x - a), the remainder will be given by P(a).
In this case, our binomial is (x + 4) or (x - (-4)). Hence, a = -4.
Then the remainder will be f(-4):
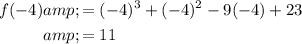
In conclusion, the remainder of the operation is 11.