Question

One-sixth of the smallest of three consecutive even

integers is three less than one-tenth the sum of the
other even integers. Find the integers. this
#49 with work pleasee

Answer 1

The three even, consecutive integers are 72, 74, and 76.

Explanation:

Let a be the first even integer.

Then the other two consecutive integers, b and c, will be represented by:

`\displaystyle b = a + 2 \text{ and } \\ \\ c = b + 2 = (a + 2) + 2 = a + 4`

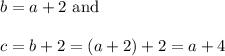
One-sixth of the smallest (that is, a) is three less than one-tenth the sum of the other two even integers (that is, b and c).

Therefore:

`\displaystyle (1)/(6) a = (1)/(10)\left( b +c\right) - 3`

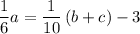
Solve for a. Substitute:

`\displaystyle (1)/(6) a =(1)/(10)\left((a+2)+(a+4)\right) - 3`

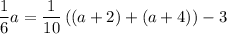
Simplify and solve for a:

`\displaystyle \begin{aligned} (1)/(6) a &= (1)/(10)(2a + 6) - 3 \\ \\ (1)/(6) a &= (1)/(5) a + (3)/(5) - 3\\ \\ -(1)/(30) a &= -(12)/(5) \\ \\ a &= 72\end{aligned}`

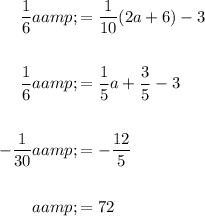
Hence, the first even integer is 72.

Therefore, the two other consecutive even integers must be 74, and 76.

In conclusion, the three even, consecutive integers are 72, 74, and 76.