Question

PLS ANSWER ASAP ILL APPRECIATE IT A LOT!!
please explain in details how u did do u could !!!

Answer 1

a) W = - 3387525.12 J = - 3.39 MJ

b) F = - 19031.04 N = - 19 KN

Step-by-step explanation:

First, we need to find the deceleration of the rocket by using the third equation of motion:

`2as = v_(f)^2 - v_(i)^2\\`

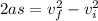
where,

a = deceleration = ?

s = distance covered = 178 m

vf = final speed = 0 m/s

vi = 284 m/s

Therefore,

`2a(178\ m) = (0\ m/s)^2 - (284\ m/s)^2\\a = (-(284\ m/s)^2))/((2)(178\ m))\\\\`

a = 226.56 m/s²

b)

Now, we can find the force by Newton's Second Law:

`F = ma\\F = (84\ kg)(-226.56\ m/s^2)\\`

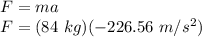
F = - 19031.04 N = - 19 KN

Here, negative sign shows braking force.

a)

For work done:

`Work = W = Fs\\W = (-19031.04\ N)(178\ m)\\`

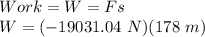
W = - 3387525.12 J = - 3.39 MJ