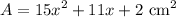Question

What is the area of a rectangle whose length is (5x + 2)cm and whose width is (3x + 1)cm?

Answer 1

`\displaystyle A = 15x^2 + 11x + 2\text{ cm}^2`

Explanation:

We are given that the length and width of a rectangle is given by:

`\ell = (5x + 2)\text{ cm} \text{ and } w = (3x+1)\text{ cm}`

And we want to find the area of the rectangle.

Recall that the area of a rectangle is given by:

`\displaystyle A = w\ell`

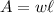
Substitute:

`A = (3x+1)(5x+2)`

Expand and simplify:

`\displaystyle \begin{aligned}A &= 5x(3x+1) + 2(3x+1) \\ &= (15x^2+5x)+(6x+2) \\ &= (15x^2)+(5x+6x)+(2) \\ &= 15x^2 + 11x +2 \end{aligned}`

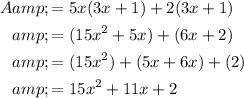
Hence, the area of the rectangle is:

`\displaystyle A = 15x^2 + 11x + 2\text{ cm}^2`