Question

In a titration experiment, 56.0 mL of an unknown concentration of H3PO4 solution is completely neutralized by 88.2 mL of 0.436 M KOH solution. Calculation the molarity of the acid. Question 11 options: 0.258 M 0.688 M 2.06 M 0.229 M 0.362 M

Answer 1

`\boxed {\boxed {\sf 0.687 \ M}}`

Step-by-step explanation:

We are asked to find the unknown concentration of the acid given the details of a titration experiment.

A formula for titration is:

`M_AV_a= M_BV_B`

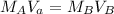
where M is the molarity of the acid of base and V is the volume of the acid or base. There are 56.0 milliliters of the acid (H₃PO₄), but the concentration or molarity of the acid is unknown. There are 88.2 milliliters of the base (KOH) with a molarity of 0.436.

`\bullet \ V_A= 56.0 \ mL\\\bullet \ M_B= 0.436 \ M \\\bullet \ V_B= 88.2 \ mL`

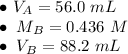
Substitute the values into the formula.

`M_A * 56.0 \ mL = 0.436 \ M * 88.2 \ mL`

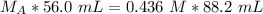
We are solving for the molarity of the acid, so we must isolate the variable
`M_A`

It is being multiplied by 56.0 milliliters and the inverse operation of multiplication is division. Divide both sides of the equation by 56.0 mL.

`\frac {M_A * 56.0 \ mL}{56.0 \ mL} = (0.436 \ M * 88.2 \ mL)/(56.0 \ mL)`

`M_A = (0.436 \ M * 88.2 \ mL)/(56.0 \ mL)`

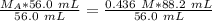
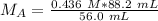
The units of milliliters/mL cancel.

`M_A = (0.436 \ M * 88.2 )/(56.0 )`

`M_A = (38.4552 )/(56.0) \ M`

`M_A = 0.6867 \ M`

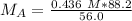
All the original measurements have 3 significant figures, so our answer must have the same. For the number we calculated, that is the thousandths place. The 7 in the ten-thousandths place tells us to round the 6 up to a 7.

`M_A= 0.687 \ M`

The molarity of the acid is 0.687 M.