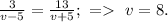Question

The speed of the current in a creek is 5 mph. A person clan kayak 3 mi upstream in the same time that it takes him to kayak 13 mi downstream. What is the speed

the person's kayak in still water?
The speed of the person's kayak in still water is

Answer 1

8

Explanation:

0) the basic formula is: L=v*t, where L - distance, v - speed/velocity; t - time;

1) if the person's speed in still water is 'v' and the speed of water is 5 (according to the condition), then the upstream speed is 'v-5' and the downstream speed is 'v+5';

2) according to the condition the upstream time and the downstream time are the same, it means t₁=t₂=t, where t₁=upstream time and t₂=downstream time;

3) according to the items above it is possible to make up the equation of the upstream travel: t(v-5)=3; ⇒ t=3/(v-5);

4) according to the items above it is possible to make up the equation of the downstream travel: t(v+5)=13; ⇒ t=13/(v+5);

5) if t=3/(v-5) and t=13/(v+5), then

`(3)/(v-5) =(13)/(v+5); \ => \ v=8.`