Question

Can someone help please! I need this last question answered

Answer 1

Answer:
`(x^2)/(16)+(y^2)/(49) = 1\\\\`

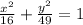
This is the same as writing (x^2)/16 + (y^2)/49 = 1

===========================================================

Step-by-step explanation:

The general equation for an ellipse is

`((x-h)^2)/(a^2)+((y-k)^2)/(b^2) = 1\\\\`

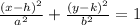
where

• (h,k) is the center
• 'a' is half the total width (along the x axis)
• 'b' is half the total height (along the y axis)

Notice how 'a' pairs with the x term, so that's why 'a' describes the horizontal width along the x axis. The horizontal width is 8 ft, which cuts in half to 4 ft. So a = 4.

The vertical length is 14 ft, which cuts in half to 7 ft. So b = 7.

The center isn't mentioned (other than the fact that the actor is located here), but I'm assuming by default it's at the origin (0,0).

With that all in mind, we then get the following:

`((x-h)^2)/(a^2)+((y-k)^2)/(b^2) = 1\\\\((x-0)^2)/(4^2)+((y-0)^2)/(7^2) = 1\\\\(x^2)/(16)+(y^2)/(49) = 1\\\\`

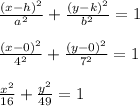
The graph is below. I used GeoGebra to make the graph.

From the graph, we can see that the horizontal width spans from x = -4 to x = 4. This is a total distance of |-4-4| = 8 feet. Similarly, the vertical length spans from y = -7 to y = 7 which is a distance of 14 feet.