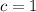Question

MY NOTES Verify that the function satisfies the three hypotheses of Rolle's Theorem on the given interval. Then find all numbers c that satisfy the conclusion of Rolle's Theorem. (Enter your answers as a comma-separated list.) f(x) = 2x2 − 4x + 3, [−1, 3

Answer 1

b)
`c=1`

Explanation:

From the question, we are told that:

Function

`F(x)=2x^2-4x+9`

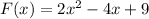
Given

Rolle's theorem states that if a function f is continuous on the closed interval [a, b] and differentiable on the open interval (a, b) such that f(a) = f(b), then f′(x) = 0 for some x with a ≤ x ≤ b.

Generally, the Function above is a polynomial that can be Differentiated and it is continuous

Where

-F(x) is continuous at (-1,3)

-F(x) Can be differentiated at (-1.3)

-And F(-1)=F(3)

Therefore

F(x) has Satisfied all the Requirements for Rolle's Theorem

Differentiating F(x) we have

`F'(x)=4x-4`

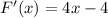
Equating F(c) we have

`F'(c)=0`

`4(c)-4=0`

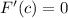
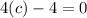
Therefore

`c=1`