Question

The New England Merchants Bank Building in Boston is 152 m high. On windy days it sways with a frequency of 0.18 Hz , and the acceleration of the top of the building can reach 1.9 % of the free-fall acceleration, enough to cause discomfort for occupants.

Required:
What is the total distance, side to side, that the top of the building moves during such an oscillation?

Answer 1

The total distance, side to side, that the top of the building moves during such an oscillation is approximately 0.291 meters.

Step-by-step explanation:

Let suppose that the building is experimenting a Simple Harmonic Motion due to the action of wind. First, we determine the angular frequency of the system (
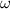`\omega`), in radians per second:

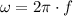`\omega = 2\pi\cdot f` (1)

Where
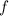`f` is the frequency, in hertz.

If we know that
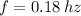`f = 0.18\,hz`, then the angular frequency of the system is:

`\omega = 2\pi\cdot (0.18\,hz)`

`\omega \approx 1.131\,(rad)/(s)`

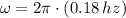
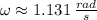
The maximum acceleration experimented by the system is represented by the following formula, of which we estimate amplitude of the oscillation:

`r\cdot g = \omega^(2)\cdot A` (2)

Where:

`r` - Ratio of real acceleration to free-fall acceleration, no unit.

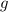`g` - Free-fall acceleration, in meters per square second.

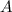`A` - Amplitude, in meters.

If we know that
`\omega \approx 1.131\,(rad)/(s)`,
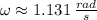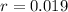`r = 0.019` and
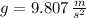`g = 9.807\,(m)/(s^(2))`, then the amplitude of the oscillation is:

`A = (r\cdot g)/(\omega^(2))`

`A = ((0.019)\cdot \left(9.807\,(m)/(s^(2)) \right))/(\left(1.131\,(rad)/(s) \right)^(2))`

`A \approx 0.146\,m`

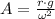
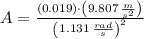
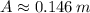
The total distance, side to side, is twice the amplitude, that is to say, a value of approximately 0.291 meters.