Answer:
work done is -2.8 × 10⁻⁶ J
Step-by-step explanation:
Given the data in the question;
mass of the pendulum m = 6 kg
Length of core = 1.7 m
Now, case1, mass is pulled aside a small distance of 7.6 cm and released from rest. so let θ₁ be the angle made by mass with vertical axis.
so, θ₁ = ( 7.6 × 10⁻² m / 1.7 m ) = 0.045 rad
In case2, mass is pulled aside a small distance of 8 cm and released from rest. so let θ₁ be the angle made by mass with vertical axis.
so, θ₂ = ( 8 × 10⁻² m / 1.7 m ) = 0.047 rad.
Now, the required work done will be;

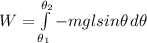
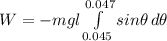
W =
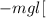 -cosθ
-cosθ
![]^(0.047)_(0.045 )](https://img.qammunity.org/qa-images/2022/formulas/physics/college/gml4n27qc0g0kz3advnnbr.png)
W = 6 × 9.8 × 1.7 × [ cos( 0.047 ) - cos( 0.045 ) ]
W = 6 × 9.8 × 1.7 × [ -2.8 × 10⁻⁸ ]
W = -2.8 × 10⁻⁶ J
Therefore, work done is -2.8 × 10⁻⁶ J