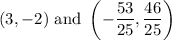Question

Find the two intersection points

(x+1)^2 +(y+2)^2 = 16

3x+ 4y = 1
Show your steps please

Answer 1

Our two intersection points are:

`\displaystyle (3, -2) \text{ and } \left(-(53)/(25), (46)/(25)\right)`

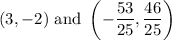
Explanation:

We want to find where the two graphs given by the equations:

`\displaystyle (x+1)^2+(y+2)^2 = 16\text{ and } 3x+4y=1`

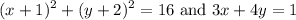
Intersect.

When they intersect, their x- and y-values are equivalent. So, we can solve one equation for y and substitute it into the other and solve for x.

Since the linear equation is easier to solve, solve it for y:

`\displaystyle y = -(3)/(4) x + (1)/(4)`

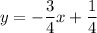
Substitute this into the first equation:

`\displaystyle (x+1)^2 + \left(\left(-(3)/(4)x + (1)/(4)\right) +2\right)^2 = 16`

Simplify:

`\displaystyle (x+1)^2 + \left(-(3)/(4) x + (9)/(4)\right)^2 = 16`

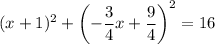
Square. We can use the perfect square trinomial pattern:

`\displaystyle \underbrace{(x^2 + 2x+1)}_((a+b)^2=a^2+2ab+b^2) + \underbrace{\left((9)/(16)x^2-(27)/(8)x+(81)/(16)\right)}_((a+b)^2=a^2+2ab+b^2) = 16`

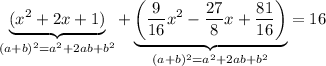
Multiply both sides by 16:

`(16x^2+32x+16)+(9x^2-54x+81) = 256`

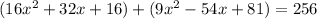
Combine like terms:

`25x^2+-22x+97=256`

Isolate the equation:

`\displaystyle 25x^2 - 22x -159=0`

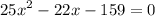
We can use the quadratic formula:

`\displaystyle x = (-b\pm√(b^2-4ac))/(2a)`

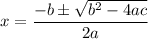
In this case, a = 25, b = -22, and c = -159. Substitute:

`\displaystyle x = (-(-22)\pm√((-22)^2-4(25)(-159)))/(2(25))`

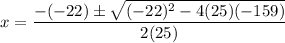
Evaluate:

`\displaystyle \begin{aligned} x &= (22\pm√(16384))/(50) \\ \\ &= (22\pm 128)/(50)\\ \\ &=(11\pm 64)/(25)\end{aligned}`

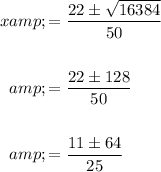
Hence, our two solutions are:

`\displaystyle x_1 = (11+64)/(25) = 3\text{ and } x_2 = (11-64)/(25) =-(53)/(25)`

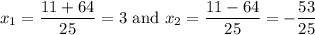
We have our two x-coordinates.

To find the y-coordinates, we can simply substitute it into the linear equation and evaluate. Thus:

`\displaystyle y_1 = -(3)/(4)(3)+(1)/(4) = -2`

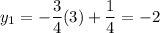
And:

`\displaystyle y _2 = -(3)/(4)\left(-(53)/(25)\right) +(1)/(4) = (46)/(25)`

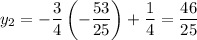
Thus, our two intersection points are:

`\displaystyle (3, -2) \text{ and } \left(-(53)/(25), (46)/(25)\right)`