Answer:
B is the Answer.
Explanation:
A transformation of a function can be represented by
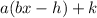
where a is the vertical stretch or compression, b is the horizontal stretch or compression. H is the shift horizontally and k is the shift vertically.
The new function has a 2 instead of 1 on the orginally function. The function will stretch since 2 is greater than 1.
B is the answer.