This question is incomplete, the complete question is;
A bat flies towards a moth at 7.1 m/s while the moth is flying towards the bat at 4.4 m/s. The bat emits a sound wave of 51.7 kHz.
After enjoying a tasty meal of the first moth, the bat goes after another moth. Flying with the same speed and emitting the same frequency, this time the bat detects a reflected frequency of 55.5 kHz. How fast is the second moth moving
Answer:
the second moth is moving at 5.062 m/s
Step-by-step explanation:
Given the data in the question;
Using doppler's effect
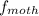 = f₀(
= f₀(
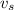 ±
±
 /
/
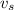 ±
±
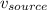 )
)
f₁ = f₀( (
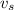 + v₂) / (
+ v₂) / (
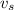 - v₁ ) )
- v₁ ) )
frequency reflected from the moth,
Now, moth is the source and the bat is the receiver
f₂ = f₁( (
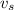 + v₁ ) / (
+ v₁ ) / (
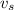 - v₂ ) )
- v₂ ) )
hence, f = f₀[ ( (
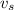 + v₁ ) / (
+ v₁ ) / (
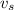 - v₂ ) ) ( (
- v₂ ) ) ( (
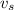 + u₂ ) / (
+ u₂ ) / (
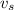 - u₁ ) )
- u₁ ) )
we know that, the velocity of sound
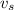 = 343 m/s.
= 343 m/s.
given that v₁ and v₂ { velocity of bat } = 7.1 m/s, f₀ = 51.7 kHz and f = 55.5 kHz.
we substitute
55.5 = 51.7[ ( ( 343 + 7.1 ) / ( 343 - 7.1 ) ) ( ( 343 + u ) / ( 343 - u ) ) ]
55.5 = 51.7[ ( 350.1 / 335.9 ) ( ( 343 + u ) / ( 343 - u ) ) ]
55.5 = 51.7[ 1.04227 ( ( 343 + u ) / ( 343 - u ) ) ]
55.5 = 53.885359 ( ( 343 + u ) / ( 343 - u ) ) ]
55.5 / 53.885359 = ( 343 + u ) / ( 343 - u )
1.02996 = ( 343 + u₂ ) / ( 343 - u )
( 343 + u₂ ) = 1.02996( 343 - u )
343 + u = 353.27628 - 1.02996u
u + 1.02996u = 353.27628 - 343
2.02996u = 10.27628
u = 10.27628 / 2.02996
u = 5.062 m/s
Therefore, the second moth is moving at 5.062 m/s