Given:
The population, P, of six towns with time t in years are given by the following exponential equations:
(i)
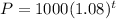
(ii)
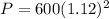
(iii)
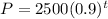
(iv)

(v)
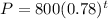
(vi)
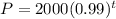
To find:
The town whose population is decreasing the fastest.
Solution:
The general form of an exponential function is:
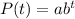
Where, a is the initial value, b is the growth or decay factor.
If b>1, then the function is increasing and if 0<b<1, then the function is decreasing.
The values of b for six towns are 1.08, 1.12, 0.9, 1.185, 0.78, 0.99 respectively. The minimum value of b is 0.78, so the population of (v) town
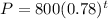 is decreasing the fastest.
is decreasing the fastest.
Therefore, the correct option is b.