Question

Lines 3x-2y+7=0 and 6x+ay-18=0 is perpendicular. What is the value of a?

1/9
9
-9
-1/9

Pls answer​

Answer 1

`\boxed{\sf a = 9 }`

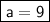
Explanation:

Two lines are given to us which are perpendicular to each other and we need to find out the value of a . The given equations are ,

`\sf\longrightarrow 3x - 2y +7=0`

`\sf\longrightarrow 6x +ay -18 = 0`

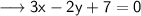
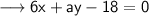
Step 1 : Convert the equations in slope intercept form of the line .

`\sf\longrightarrow y = (3x)/(2) +( 7 )/(2)`

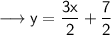
and ,

`\sf\longrightarrow y = -(6x )/(a)+(18)/(a)`

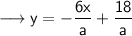
Step 2: Find the slope of the lines :-

Now we know that the product of slope of two perpendicular lines is -1. Therefore , from Slope Intercept Form of the line we can say that the slope of first line is ,

`\sf\longrightarrow Slope_1 = (3)/(2)`

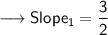
And the slope of the second line is ,

`\sf\longrightarrow Slope_2 =(-6)/(a)`

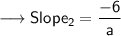
Step 3: Multiply the slopes :-

`\sf\longrightarrow (3)/(2)* (-6)/(a)= -1`

Multiply ,

`\sf\longrightarrow (-9)/(a)= -1`

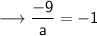
Multiply both sides by a ,

`\sf\longrightarrow (-1)a = -9`

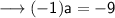
Divide both sides by -1 ,

`\sf\longrightarrow \boxed{\blue{\sf a = 9 }}`

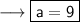
Hence the value of a is 9 .