Answer:
a) v = 8,878 km / h, θ’= 238.8º, b) t = 1890.9 s
Step-by-step explanation:
a) In this exercise we must find the resulting speed of the boat.
Let's use trigonometry to break down the speed of the boat (v1)
cos 225 = v₁ₓ / v₁
sin 225 = v_{1y} / v₁
v₁ₓ = v₁ soc 225
v_{1y} = v₁ sin 225
v₁ₓ = 6.5 cos 225 = -4.596 km / h
v_{1y} = 6.5 sin 225 = -4.596 km / h
to find the velocity we add each component
vₓ = v₁ₓ
vₓ = - 4,596 km / h
v_y = v_{1y} + v₂
v_y = -4.596 - 3
v_y = - 7,596 km / h
Now let's compose the speed
Let's use the Pythagorean theorem for the module
v =
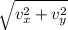
v = Ra 4.596² + 7.596²
v = 8,878 km / h
Let's use trigonometry for the direction
tan θ = v_y / vₓ
θ = tan⁻¹ v_y / vₓ
θ = tan⁻¹ (
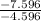 )
)
θ = 58.8º
measured from the positive side of the x-axis
θ'= 180 + 58.8
θ’= 238.8º
b) Let's reduce the river width to the SI system
x = 1.5 miles (1,609 km / 1 mile) = 2,414 km
to cross the river the speed is on the x axis which is the width of the river
v = x / t
t = x / v
t = 2.414 /4.596
t = 0.525 h
let's reduce to the SI system
t = 0.525 h (3600 s / 1h)
t = 1890.9 s