Question

Please help!!

Without graphing, explain how you know the equation
y = - 1/2x^2 - 7 will be compressed
(compared to the parent function of y=x^2).

Answer 1

The absolute value of the quadratic term,
`\left | -(1)/(2) \right |` is less than 1

Explanation:

The given function is y = (-1/2)·x² - 7

The parent function is y = x²

The vertical compression or stretching of a quadratic function is given by the value of the coefficient, a, of the quadratic term, x² of a quadratic function, a·x²

A quadratic function is vertically compressed if the coefficient,
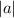`\left | a \right |` < 1.

In the given function, y = (-1/2)·x² - 7, the absolute value of the coefficient of the quadratic term,
`\left | -(1)/(2) \right |` < 1, therefore, the equation, y = (-1/2)·x² - 7, will be vertically compressed compared to the parent function, y = x².