Question

The sum of Ivy's and Audrey's ages is 27. Nine years ago, Ivy was

twice as old as Audrey. How old is each now?

Answer 1

Ivy is 15 years old and Audrey is 12 years old.

Explanation:

Let Ivy's age be
`i` and Audrey's age be
`a`.

Since the sum of their ages is 27, we can write the equation
`i+a=27`.

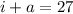Next, we'll write a second equation from the fact that 9 years ago Ivy was twice as old as Audrey. Nine years ago, Ivy and Audrey's ages were
`i-9` and
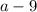`a-9`, respectively. Therefore, we have
`i-9=2(a-9)`

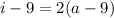
Let's isolate
`i` by adding 9 to both sides:

`i=2(a-9)+9`

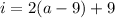
Distribute:

`i=2a-18+9,\\i=2a-9`

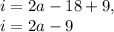
Now substitute
`i=2a-9` into our first equation:

`2a-9+a=27,\\3a-9=27, \\3a=36, \\a=\boxed{12}`

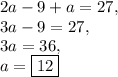
Therefore, Ivy's age must be:

`i+12=27,\\i=27-12=\boxed{15}`

Thus, Ivy must be 15 years old and Audrey must be 12 years old.