Given:
The function is:
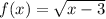
To find:
The inequality that is used to find the domain of the given function.
Solution:
We have,
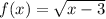
We know that the radical functions are defined for only positive values of radicand.
It means the given function is defined if the value of (x-3) is greater than or equal to 0.
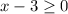
Adding 3 on both sides, we get
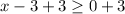
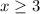
The inequality
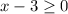 is used to find the domain of the given function and the domain of the given function is
is used to find the domain of the given function and the domain of the given function is
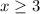 .
.
Therefore, the correct option is B.